31 Find the Vertex of y = x 24x32 Parabolas have a highest or a lowest point called the Vertex Our parabola opens up and accordingly has a lowest point (AKA absolute minimum) We know this even before plotting y because the coefficient of the first term, 1 , is positive (greater than zero)1Express the Quadratic Function in Standard Form 2Find it's Vertex and its x and y intercept(s) Standard form of equation , (h,k)=(x,y) coordinates of the vertex, A is a coefficient that affects the slope or steepness of the curve f(x)=2x^24x3Free factor calculator Factor quadratic equations stepbystep

Putting Functions Into Vertex Form
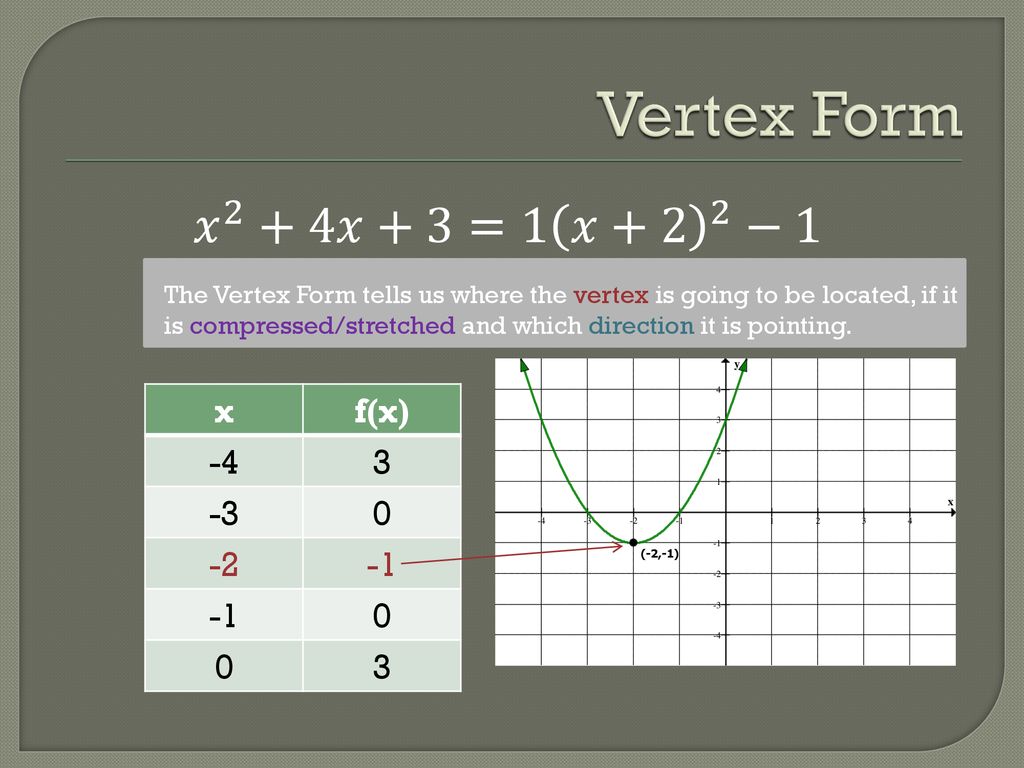
F(x)=x^2-4x+3 in vertex form
F(x)=x^2-4x+3 in vertex form-Q Write y = x 2 4x 1 in vertex form What is the axis of symmetry of y= 2x 2The above quadratic is in the form of y = a(x h) 2 k Here, the vertex is (h, k) Solved Examples Example 1 Write the following quadratic function in vertex form and sketch the parabola y = x 2 4x 3 Solution Step 1 In the quadratic function given, the coefficient of x



Graph Linear Quadratic Functions Solutions Examples Lessons Worksheets Activities
Answer The values p and q are xintercepts of the graph of the function f (x) = a (x – p) (x – q) Question 2 WRITING Explain how to find the maximum value or minimum value of a quadratic function when the function is given in intercept form Answer The intercept form is f (x) = a (x – p) (x – q) x = (pq)/2Review Vertex and Intercepts of a Quadratic Functions The graph of a quadratic function of the form f(x) = a x 2 b x c is a vertical parabola with axis of symmetry parallel to the y axis and has a vertex V with coordinates (h , k), x intercepts when they exist and a y intercept as shown below in the graph When the coefficient a is positive the vertex is the lowest point in the4 ← is in vertex form with vertex = (2, 4 ) This is a vertical parabola, opening upwards and is symmetrical about the vertex The axis of symmetry is a vertical line with equation x = 2 Answer from monacelliowlrlu SHOW ANSWER x = 4/3 Stepbystep explanation 3 (x 2)2 4
Is addition and one when it is subtraction All equations of the form a x 2 b x c = 0 can be solved using the quadratic formulaNote, however, that the result of this manipulation, \(f(x) = x^2 4x 7\), is not as useful as vertex form, as it is difficult to identify the transformations required to draw the parabola represented by the equation \(f(x) = x^2 4x 7\) It's reallySuppose a parabola has a vertex (4,7) and also passes through the point (3,8) Write the equation of the parabola in vertex form f(x)=a(xh)^2k I believe h=4 k=7 Not sure what to do from here asked by Lee on October 29, 12
Find the Vertex f (x)=x^24x3 f (x) = x2 − 4x 3 f ( x) = x 2 4 x 3 Rewrite the equation in term of x x and y y f (x) = x2 −4x3 f ( x) = x 2 4 x 3 Rewrite the equation in vertex form Tap for more steps Complete the square for x 2 − 4 x 3 x 2 4 x 3 Tap for more steps Use the form a x 2 b x c a x 2 b x cVideo Transcript we have this equation of a proble and we want to put it into Vertex form first out of the two terms right there when a factor A negative, too That would give us X squared minus six X And then I'm gonna put the minus 11 in the back So we need to complete the square by adding something here and will also be compensating for(x 3) = ±



Finding The Vertex Of A Parabola In Standard Form Video Khan Academy




Calameo Algebra 2 Mcgraw Hill
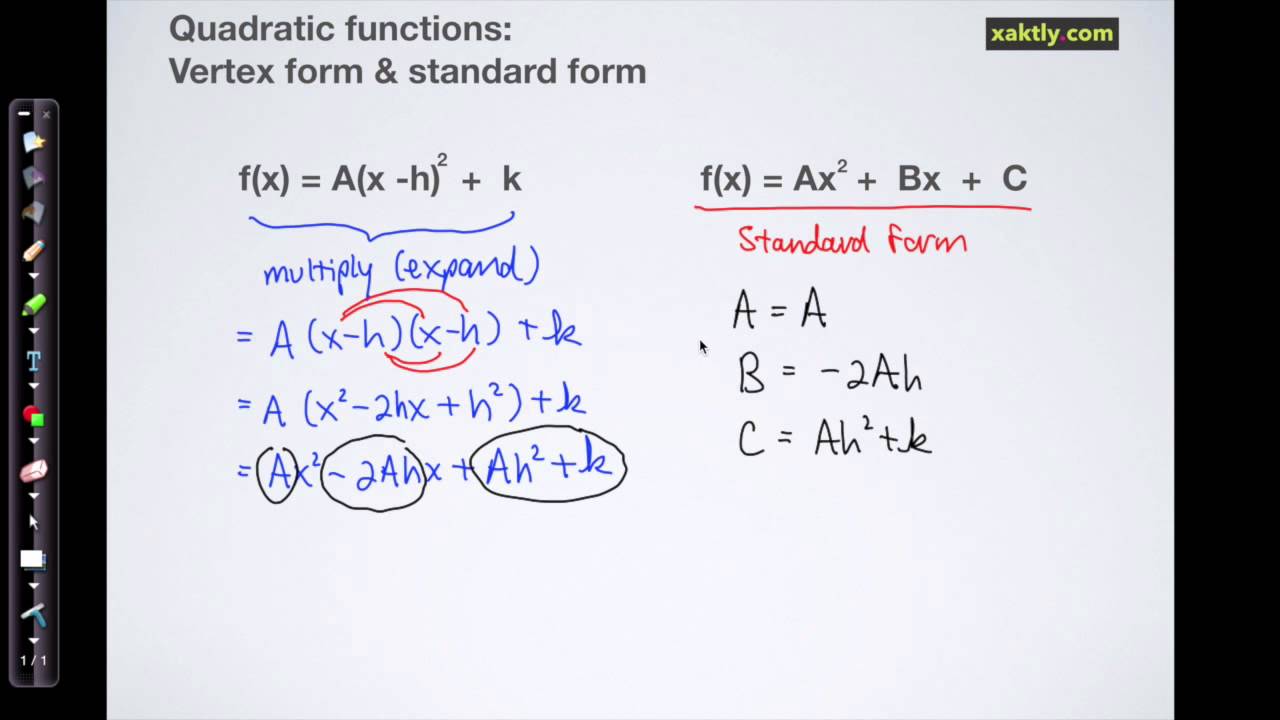
Express {eq}f(x) = x^2 4x 3 {/eq} in standard form and find its maximum or minimum value Completed square form vertex, and factored forms to model aGiven quadratic function f(x) = x^2 2x 6 Express f(x) in standard form Also find the vertex, xintercept, and yintercept of the function Given quadratic function 4x^2 40x 107 Express f(x) in standard form Find the vertex, xintercept, and yintercept Find the Maximum or Minimum value of the function f(x) = 2x^2 4x 3The formula for the vertex form of a parabola is f(x) = a(x h) 2 k where a = vertical stretch or shrink of the parabola and (h, k) are the (x, y) coordinates of the vertex of the parabola h = the xcoordinate and k = the ycoordinate



Solved 5 The Graph Of The Absolute Value Parent Function 6 Which Function Has A Vertex At 1 3 Course Hero




Alg2b Exam Review 15 16 Quadratic Equation Elementary Mathematics
The graph of a quadratic function is called a Q The quadratic parent function Q What is the axis of symmetry of the given function?A quadratic function in the form f (x) = ax2 bxx f ( x) = a x 2 b x x is in standard form Regardless of the format, the graph of a quadratic function is a parabola The graph of y=x2−4x3 y = x 2 − 4 x 3 The graph of any quadratic equation is always a parabolaSolvevariablecom makes available simple info on convert to vertex form calculator, substitution and logarithmic and other algebra subjects When you need help on subtracting rational expressions or mathematics courses, Solvevariablecom is the right destination to go to!




F X X2 12x




Car Nationals Ofmc Assessment See How To Solve It At Qanda
The vertex form is a special form of a quadratic function From the vertex form, it is easily visible where the maximum or minimum point (the vertex) of the parabola is The number in brackets gives (trouble spot up to the sign!) the xcoordinate of the vertex, the number at the end of the form gives the ycoordinateSolve Step Graph f(x) = x 2 6x 8 Complete the square * f(x) = x 2 6x 99 8 Take half of 6 and square it f(x) = (x 3) 21 (x 3) 2 = x 2 6x 9Vertex (3,1) Vertex is (h,k)This means the vertex is shifted 3 units left and 1 unit down from the origin *Check out completing the square for help with this stepA free online vertex form calculator can convert vertex form to the standard form of a parabola If you want to know how to change the vertex to standard form, let's start!



How Do You Write F X X 2 4x In Vertex Form Socratic



Ppt Completing Square Of A Quadratic Function Powerpoint Presentation Id
You can put this solution on YOUR website!About Graphing Quadratic Functions Quadratic function has the form $ f(x) = ax^2 bx c $ where a, b and c are numbers You can sketch quadratic function in 4 steps I will explain these steps in following examplesThe vertex of a quadratic equation in vertex form is (h,k), so our vertex is (3,22) Notes Right now our quadratic equation, y=2x 2 12x4 is in standard form We want to get it into vertex form To do this, we are going to use the method of completing the square




Finding The Vertex Of A Parabola In Standard Form Video Khan Academy




The First Steps In Writing F X 3x2 24x 10 In Vertex Form Are Shown F X 3 X2 8x 10 Brainly Com
This algebra video tutorial explains how to convert a quadratic equation from standard form to vertex form and from vertex form to standard form This videoFree functions vertex calculator find function's vertex stepbystep This website uses cookies to ensure you get the best experience By using this website, you agree to our Cookie PolicyMultiply the inequality by 1 to make the coefficient of the highest power in − x 2 4 x 1 positive Since − 1 is negative, the inequality direction is changed x^ {2}4x1<0 x 2 − 4 x − 1 <




Vertex Axis Of Symmetry Of A Parabola Video Khan Academy



Writing Quadratic Equations Interactive Worksheet By Madeline Frazier Wizer Me
Example 3 Find the vertex form of the quadratic function below Solution Factor out \,3 among the xterms The coefficient of the linear term inside the parenthesis is \,1 Divide it by 2 and square it Add that value inside the parenthesis Now, figure out how tof(x)=(x2)^23 or (x(2))^23 Vertex form of equation is f(x)=a(xh)^2k Here, we have f(x)=x^24x1, hence we have a=1 So for converting to this form, we complete the square using (xa)^2=x^22axa^2 for which we have to identify a and then add and subtract a^2 Hence, f(x)=ul(x^22xx2xx x2^2)2^21 =(x2)^241 =(x(2))^23ID A 1 FIFC8 Vertex Form of a Quadratic Answer Section 1 ANS 2 REF ai 2 ANS 1 x2 −12x7 x2 −12x36−29 (x−6)2 −29 REF 0815ai 3 ANS 1 y=x2 24x144−18−144 y=(x12)2 −162 REF ai




Graphing Parabolas




Answered In Exercises 19 To 28 Use The Vertex Bartleby
0 To solve the inequality, factor the left hand sideX^ {2}4x7=0 x 2 − 4 x − 7 = 0 All equations of the form ax^ {2}bxc=0 can be solved using the quadratic formula \frac {b±\sqrt {b^ {2}4ac}} {2a} The quadratic formula gives two solutions, one when ±Vertex form Vertex form is another form of a quadratic equation The standard form of a quadratic equation is ax 2 bx c The vertex form of a quadratic equation is a (x h) 2 k where a is a constant that tells us whether the parabola opens upwards or downwards, and (h, k) is the location of the vertex of the parabola




Graphing Parabolas
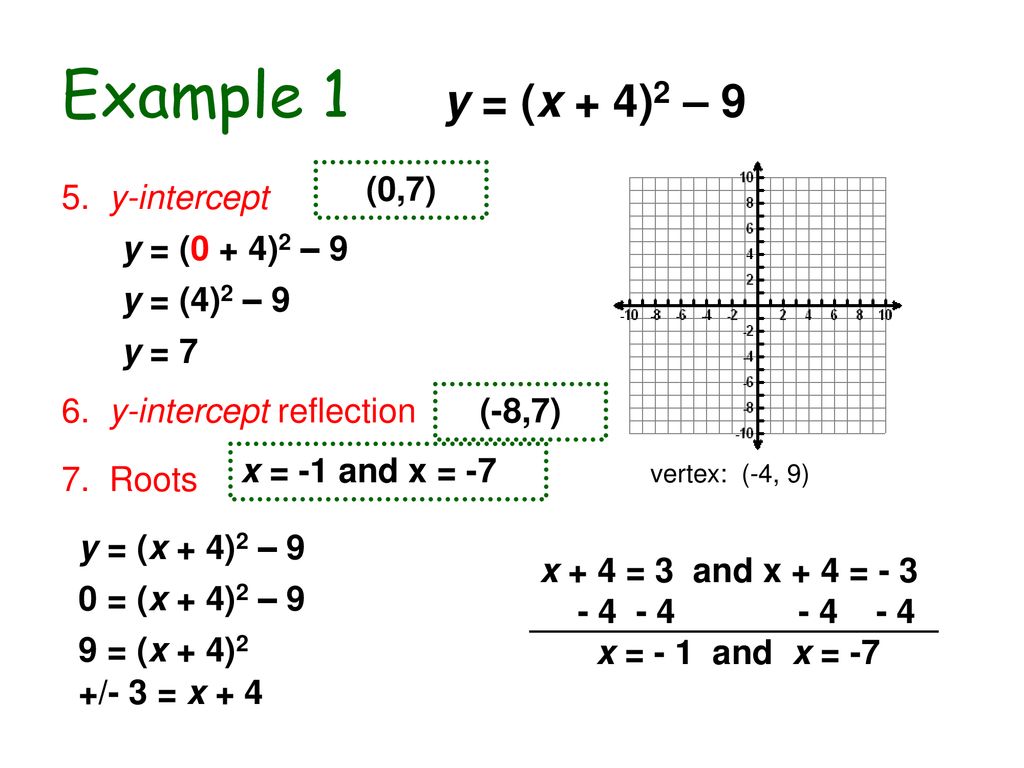



Using The Vertex Form Of Quadratic Equations Ppt Download
Select a few x x values, and plug them into the equation to find the corresponding y y values The x x values should be selected around the vertex Tap for more steps Replace the variable x x with 0 0 in the expression f ( 0) = − 4 ( 0) 2 8 ( 0) − 3 f ( 0) = 4 ( 0) 2 8 ( 0) 3 Simplify the resultB Standard form of a quadratic function and vertex Any quadratic function can be written in the standard form f(x) = a(x h) 2 k where h and k are given in terms of coefficients a, b and c Let us start with the quadratic function in general form and complete the square to rewrite it in standard form Given function f(x) f(x) = ax 2 bx c factor coefficient a out of the terms in x 2f (x) = 3 (x 2)²




Converting A Quadratic Function From Standard Form To Vertex Form Completing The Square Youtube




Answered Metro By T Mobile 5 42 Am 39 Back Bartleby
Q What is the constant that should be added to the expression to complete the square x 2 16x Q What is the vertex of y=x 2 4x3?View this answer The given equation is y =x24x−3 y = x 2 4 x − 3 To convert this into the vertex form, we have to complete the squares Adding 3 3 on both sidesMove the constant over to achieve vertex form is the final answer with vertex at (1,7) Note that the formula is try this shortcut after you have mastered the steps Make sure you recognize that this formula gives you an x and y coordinate for the vertex and that each coordinate of the pair is fraction in the formula




The Vertex Form Of The Equation Of A Parabola Is Y 3 X 4 2 13 What Is The Standard Form Of The Brainly Com
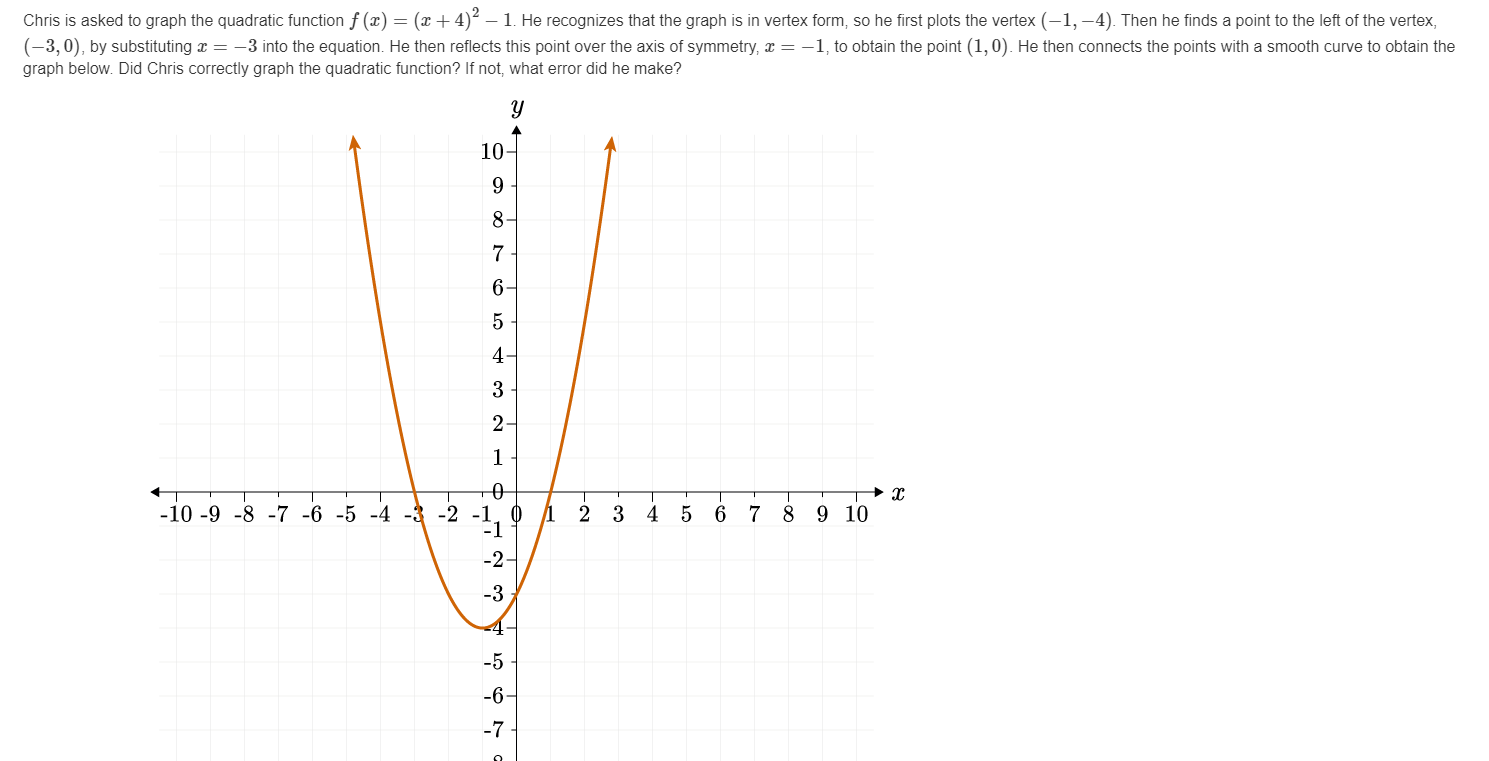



Chris Is Asked To Graph The Quadratic Function F X Chegg Com
To Complete the Square on \( x^24x \) we must take half of 4 which is 2 and compute \( (x2)^2 = x^24x4\) 2) We therefore add 8 to both sides (as 2*4=8 to the right side) to get \(y6 8=2(x^24x4) \) This Completes The Square We rewrite \( y2=2(x2)^2 \) 3) Subtracting 2 yields the Vertex Form \( f(x) = 2(x2)^22 \)The a in the vertex form is the same a as in y = ax^2 bx c Your equation is f(x) = x^24x3 We convert to the vertex form by completing the square Step 1 Move the constant to the other side f(x)3 = x^24x Step 2 Square the coefficient of x and divide by 4 (4)^2/4 = 16/4 = 4 Step 3 Add this value to each side f(x)34= x^24xA quadratic function is given f (x) = x^2 4x 4 Express the quadratic function in standard form Find its vertex and its x and yintercept (s) (Enter your answers as ordered pairs separated by commas >) A quadratic function is given f (x) = 2x^2 x 6 Express the quadratic function in standard form




Ppt Completing Square Of A Quadratic Function Powerpoint Presentation Id
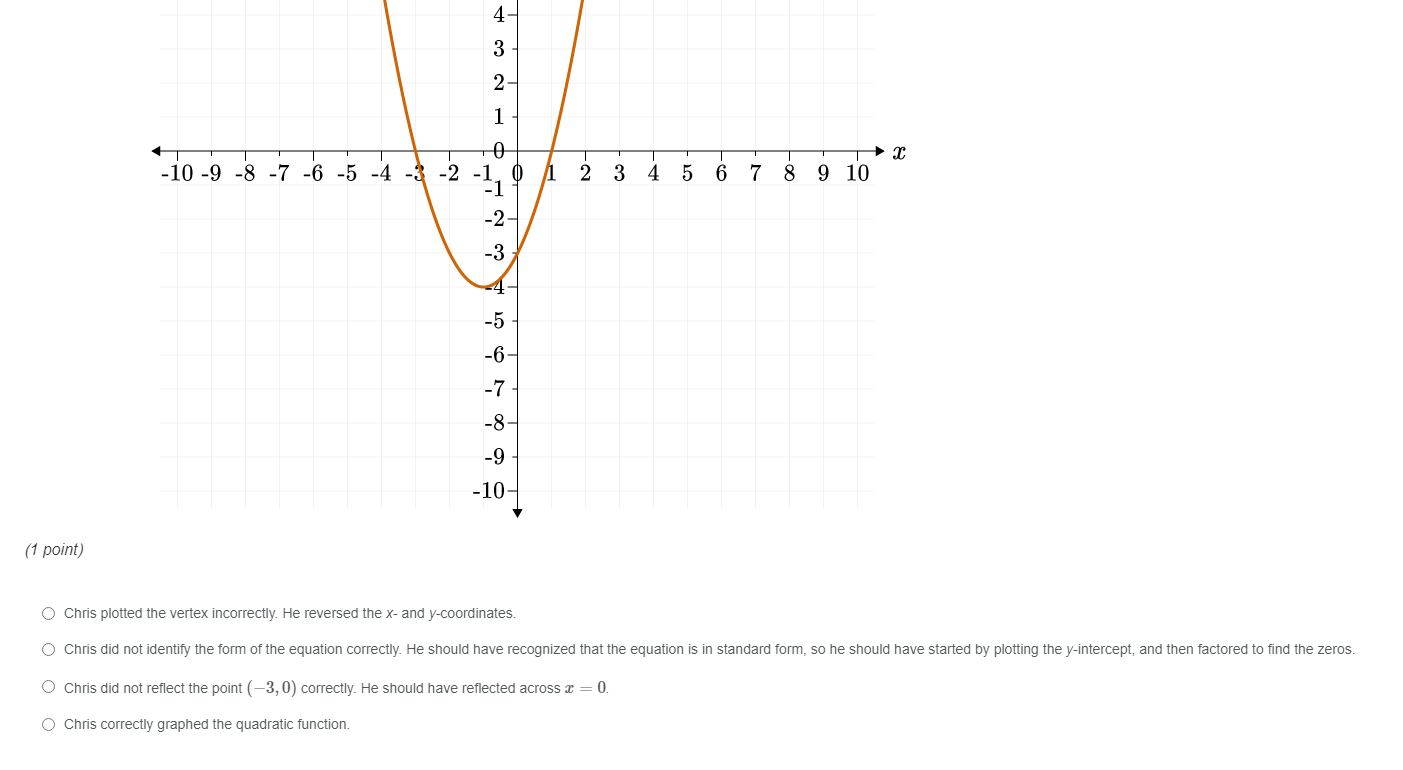



Chris Is Asked To Graph The Quadratic Function F X Chegg Com
To find the vertex form of the parabola, we use the concept completing the square method Vertex form of a quadratic function y = a(x h) 2 k In order to find the maximum or minimum value of quadratic function, we have to convert the given quadratic equation in the above formIf given the equation y = 3 (x 5) 2 4, what is the vertex of the parabola?Sqrt(2) To sketch the graph of f we shift the graph of y = x 2 three units to the right and two units down If the coefficient of x 2 is not 1, then we must factor this coefficient from the x 2 and x terms before proceeding Example 4 Write f(x) = 2x 2 2x 3 in standard form and find the vertex of the graph
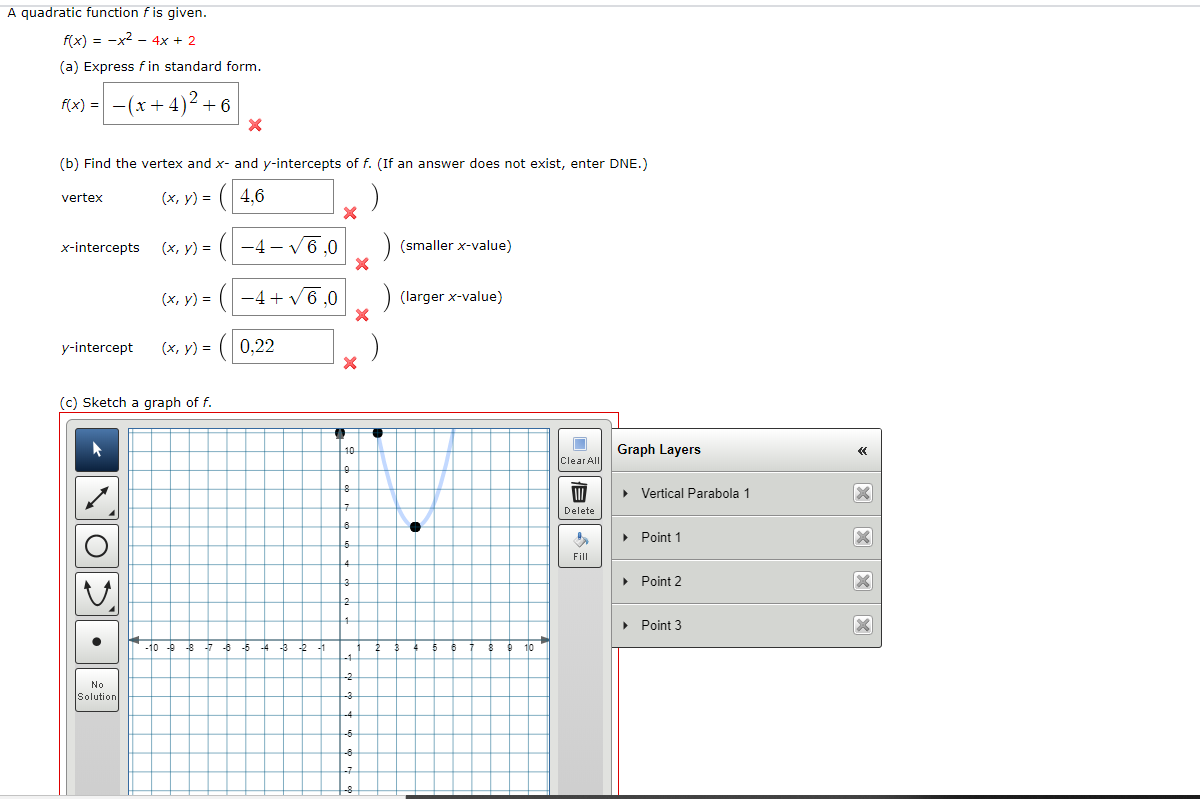



A Quadratic Function F Is Given F X X2 4x 2 Chegg Com



Solved 1 Write The Function F X 2x 2 12x 3 In Vertex Form 2 Verify Your Answer To Question 1 Above By Expanding And Simplifying To Standard For Course Hero
One formula works when the parabola's equation is in vertex form and the other works when the parabola's equation is in standard form Standard Form If your equation is in the standard form $$ y = ax^2 bx c $$ , then the formula for the axis of symmetry is $ \red{ \boxed{ xWrite an equation in vertex form m = a ( x – h) 2 K Now, expand the square formula m = a ( x 2 y 2 2 h x) K Multiply the inner side or bracket a x 2 a y 2Concavity Describe the concavity of the parabola for each function Write "concave up" or "concave down"




What Is The Vertex Form Of Y X 2 16x 71 With Steps Please Brainly Com
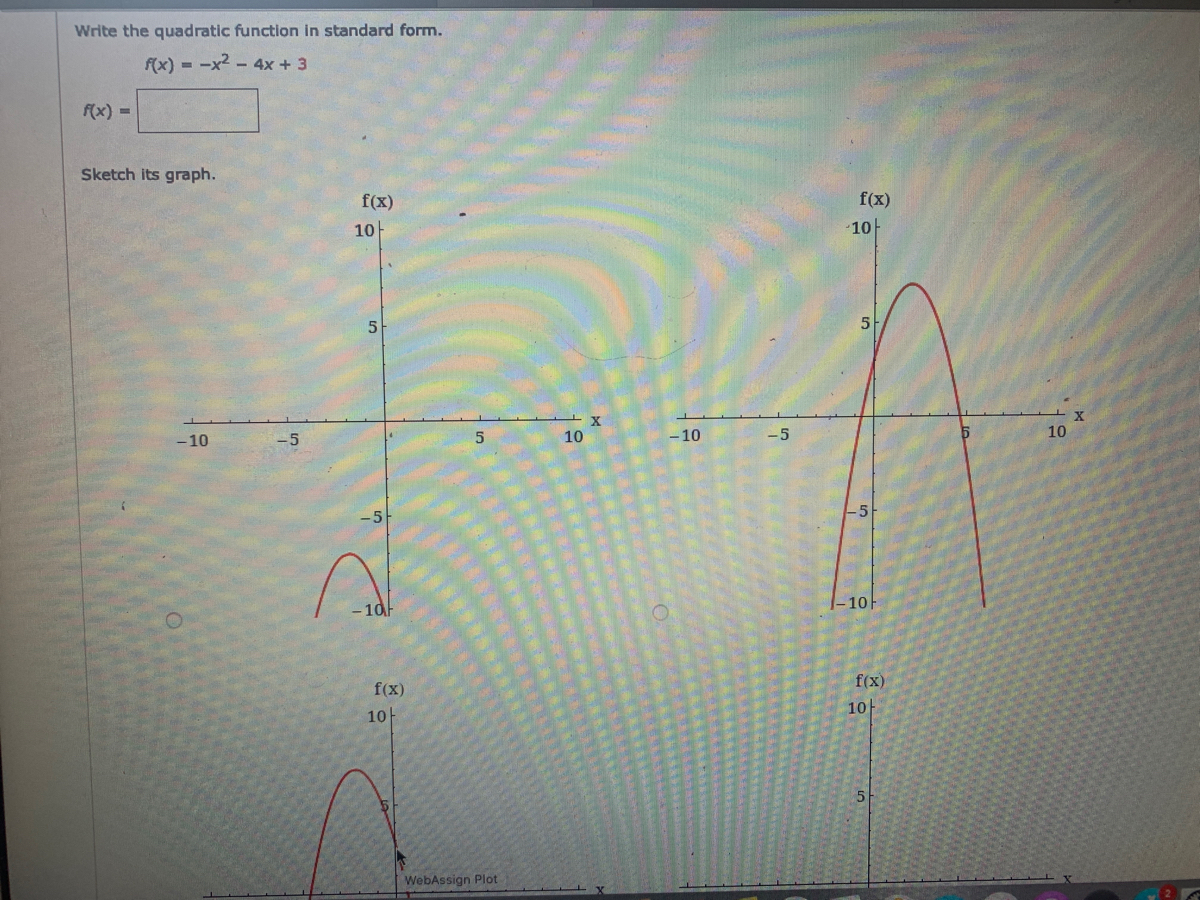



Answered Write The Quadratic Function In Bartleby
If it's negative, the parabola opens downward In the function f(x) = 2x^2 5x 4, the coefficient of x^2 is positive, so the parabola opens upward Next, find the x value of the vertex by solving b/2a, where b is the coefficient in front of x and a is the coefficient in front of x^2 In the function f(x) = 2x^2 5x 4, b = 5 and a = 2Solving x 24x21 = 0 directly Earlier we factored this polynomial by splitting the middle term let us now solve the equation by Completing The Square and by using the Quadratic Formula Parabola, Finding the Vertex 31 Find the Vertex of y = x 24x21 Parabolas have a highest or a lowest point called the VertexSqrt(2) x = 3 ±




Putting Functions Into Vertex Form




How To Find The Vertex Of A Quadratic Equation 10 Steps
Mar 31, 12 #1 I need to express the function f (x)= 5x 2 x 2 In Vertex Form I have attempted to do it two different ways, but am unsure if I am doing it correctly Attempt 1 Perfect Square f (x)= 5x 2 x 2 = 5 ( x 2 4x ) 2The yintercept is the value of y when x = 0 to find that, simply replace x with 0 in your equation to get y = x^2 4x 3 becomes y = 0 0 3 which becomes y = 3 as confirmed by the graph to plot the graph, you simply take values of x and solve for y and then plot the value of the (xWrite y = 2x^2 8x 3 in vertex form 2 See answers altavistard altavistard We'll use completing the square to rewrite y = 2x^2 8x 3 in vertex form y = 2(x^2 4x ) 3 Halve the coefficient of x (that is, halve 4), obtaining 2, and then square 2




7 1 Graphs Of Quadratic Functions In Vertex Form Pdf Free Download



Graphing Y A X H K Youtube
🔴 Answer 1 🔴 on a question The axis of symmetry for a function in the form f(x) = x2 4x − 5 is x = −2 what are the coordinates of the vertex of the graph?F (x) = x 2 4x 12 Q What is the yintercept of Q Write y = x 2 4x 1 in vertex formClick here 👆 to get an answer to your question ️ what is the vertex and equation for axis of symmetry of f(x)= x^2 4x3 loldude1266 loldude1266 05/30/19 Mathematics Middle School answered What is the vertex and equation for axis of symmetry of f(x)= x^2 4x3 2




7 1 Graphs Of Quadratic Functions In Vertex Form Quadratic Flip Ebook Pages 1 39 Anyflip Anyflip



Convert To Vertex Form With A Negative X Squared Youtube
The line of symmetry runs through the vertex so the x of the vertex is b/(2a) and the equation of the line of algebra Write the vertex form of a parabola that satisfies the conditions given Then write the equation in the form y=ax^2bxc Vertex (5,3) and a=2 You can view more similar questions or ask a new question



Forms Features Of Quadratic Functions Video Khan Academy



Plot A Graph For The Equation Y X 2 4x 1
/1000px-Parabola_features-58fc9dfd5f9b581d595b886e.png)



How To Find Quadratic Line Of Symmetry




7 1 Graphs Of Quadratic Functions In Vertex Form Pdf Free Download




Graph Linear Quadratic Functions Solutions Examples Lessons Worksheets Activities




Oirection Write The Following See How To Solve It At Qanda




Algebra 2 4 1 Graph Quadratic Functions In Standard Form Pdf Free Download



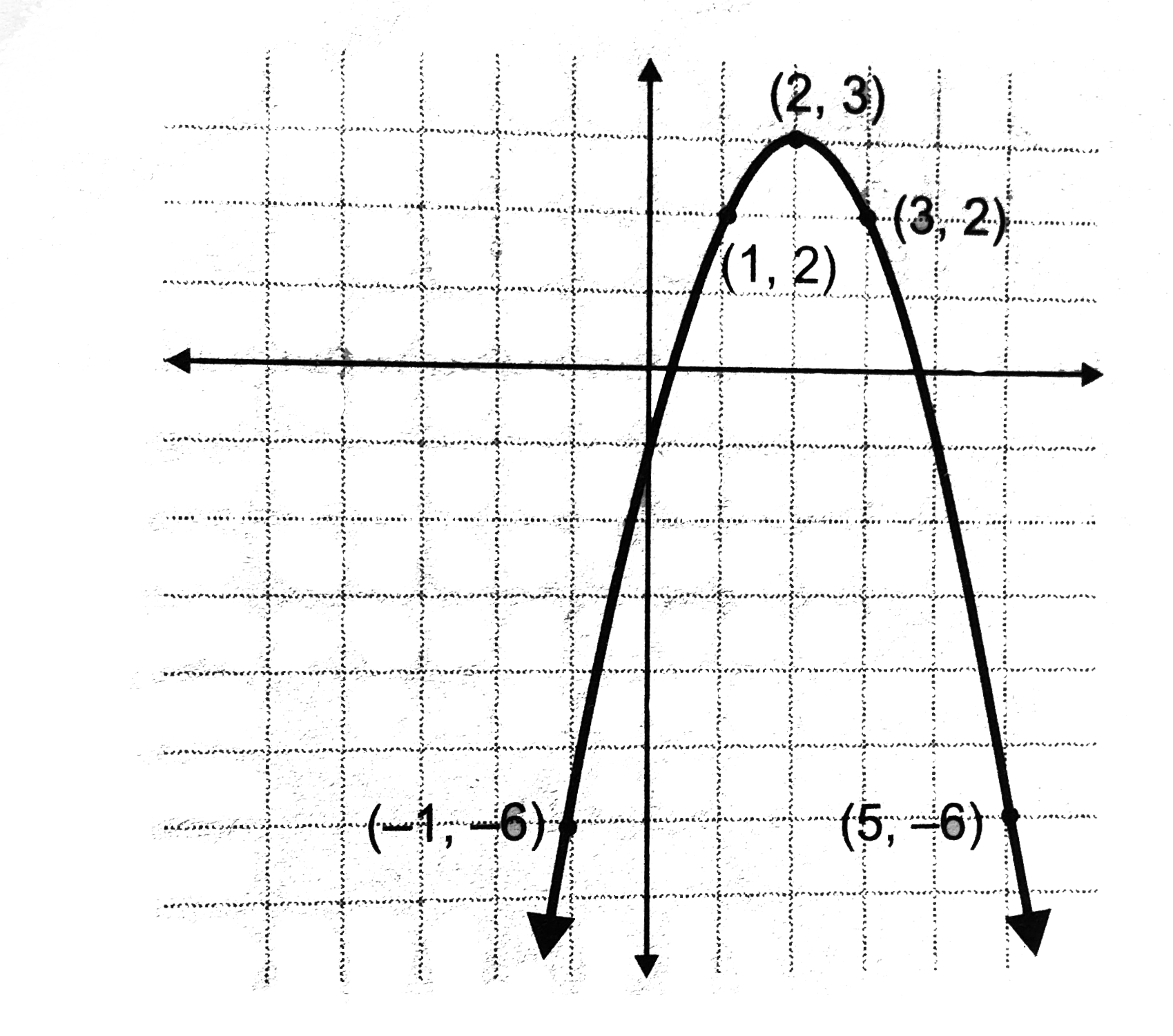
Y Classzone
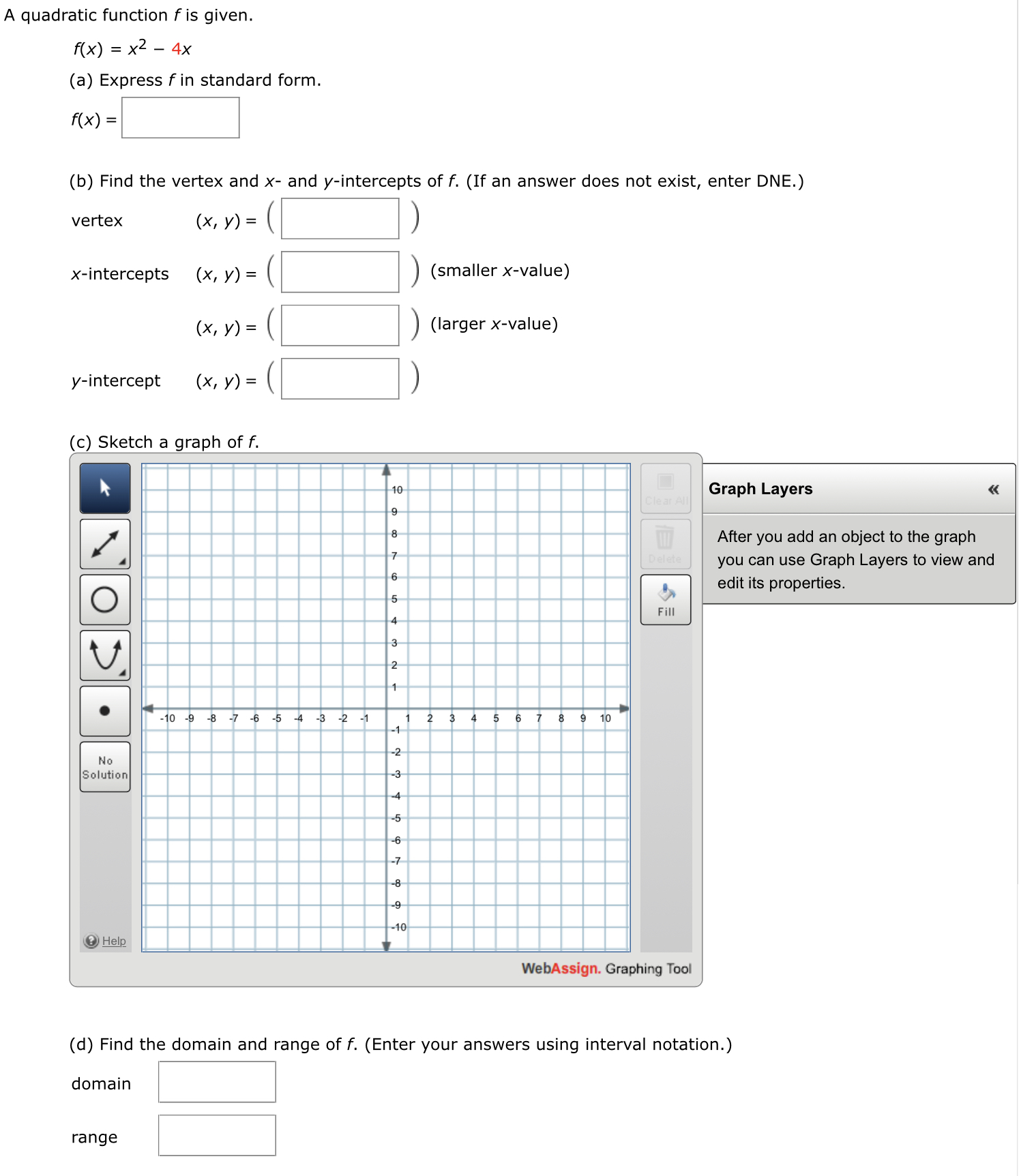



Answered A Quadratic Function F Is Given F X Bartleby
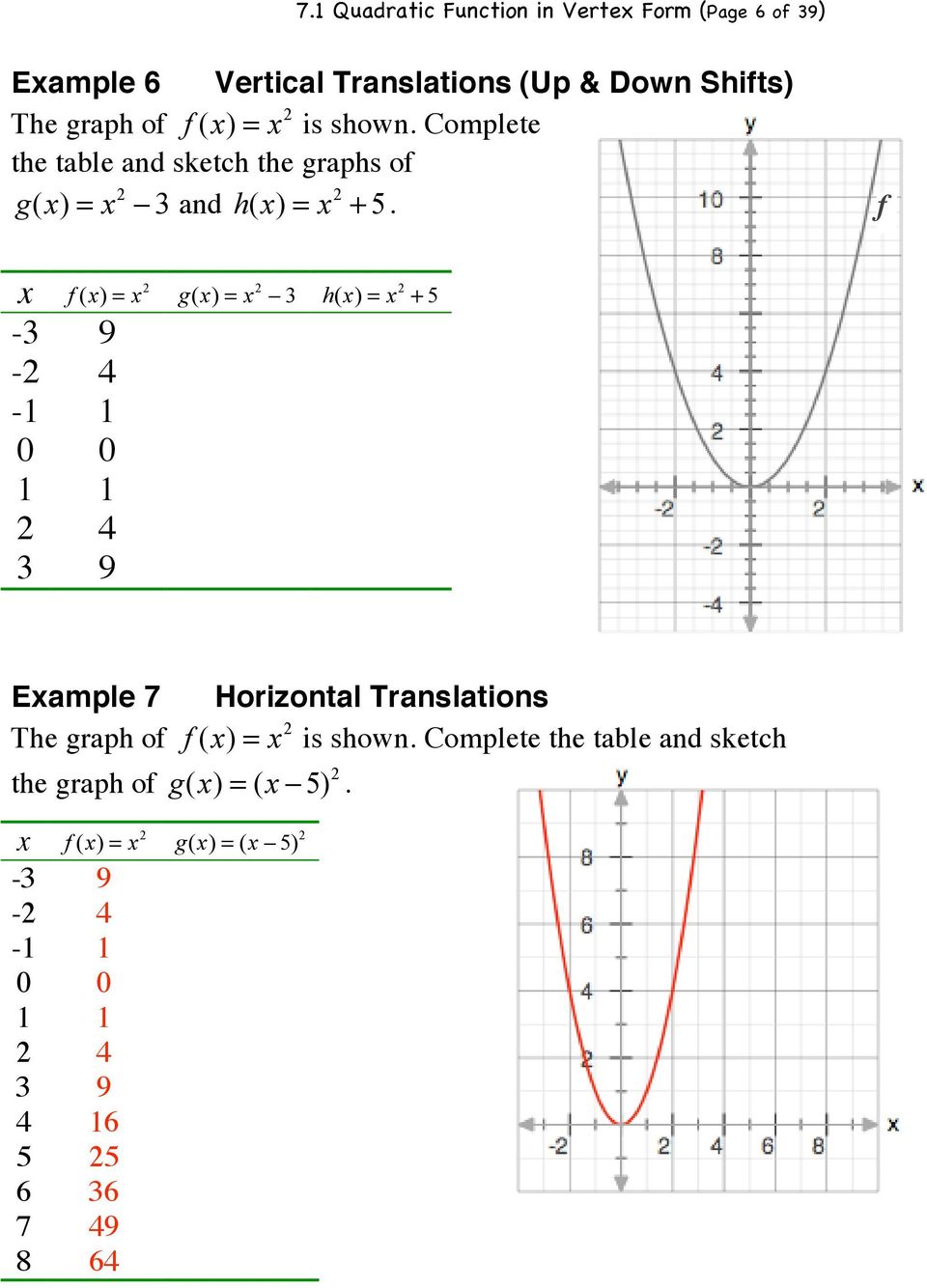



7 1 Graphs Of Quadratic Functions In Vertex Form Pdf Free Download
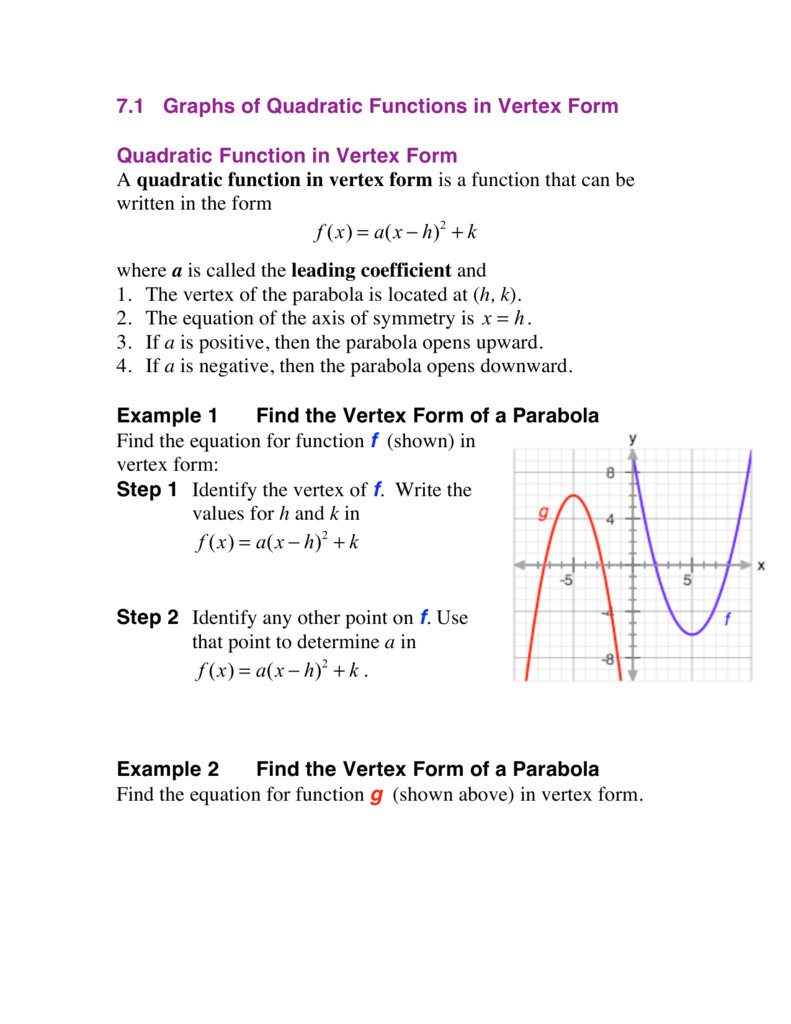



F X A X H 2 K F X A X H 2 K F X A X H 2 K




Quadratic And Vertex Form Adventure Crossword Wordmint




Representations Of Quadratic Functions Normal Shape Vertex Shape And Factor Representations Of Quadratic Fu Quadratic Functions Quadratics Math Methods
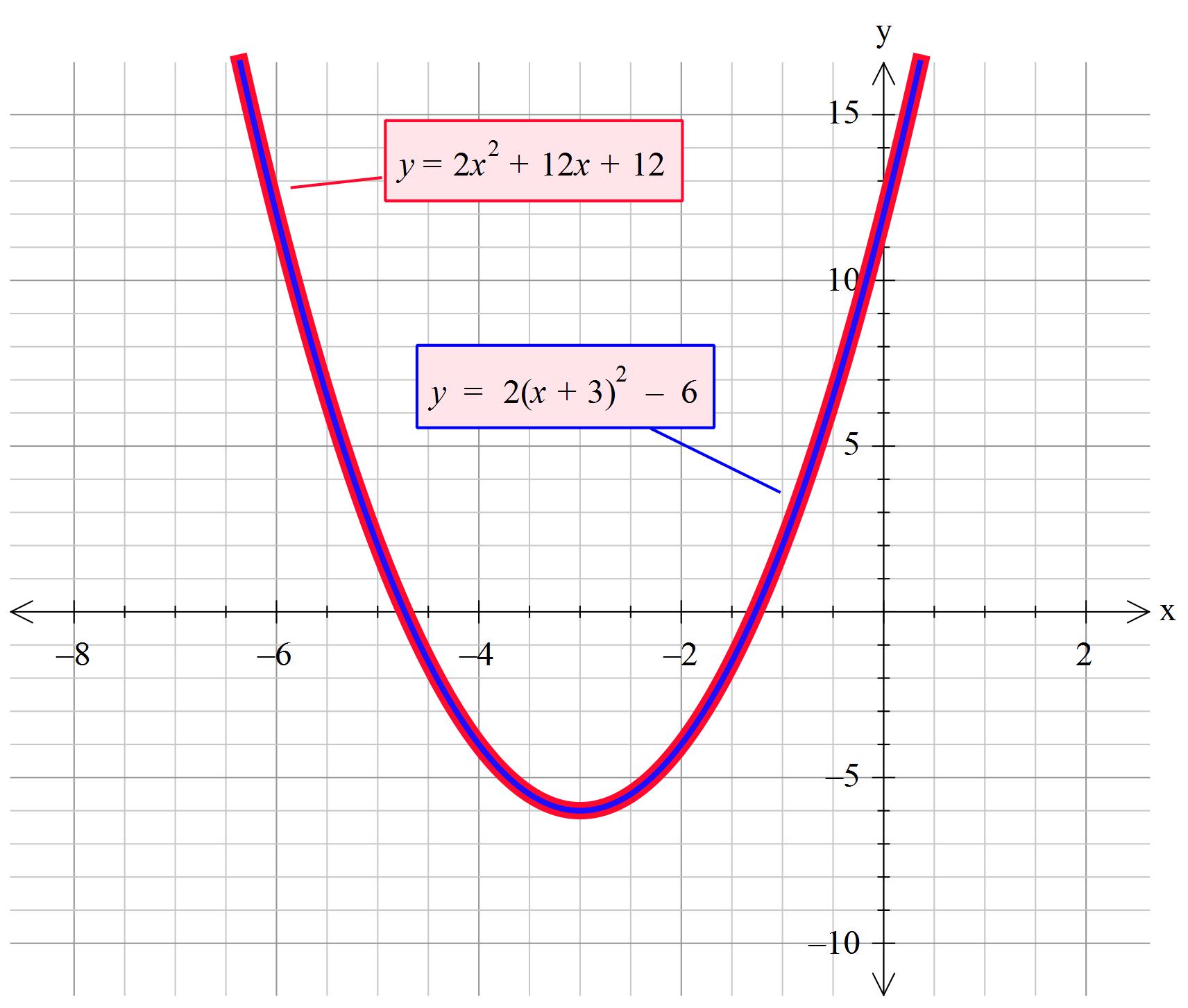



How Do You Write F X 2x 2 12x 12 In Vertex Form Socratic



7 1 Graphs Of Quadratic Functions In Vertex Form Pdf Free Download
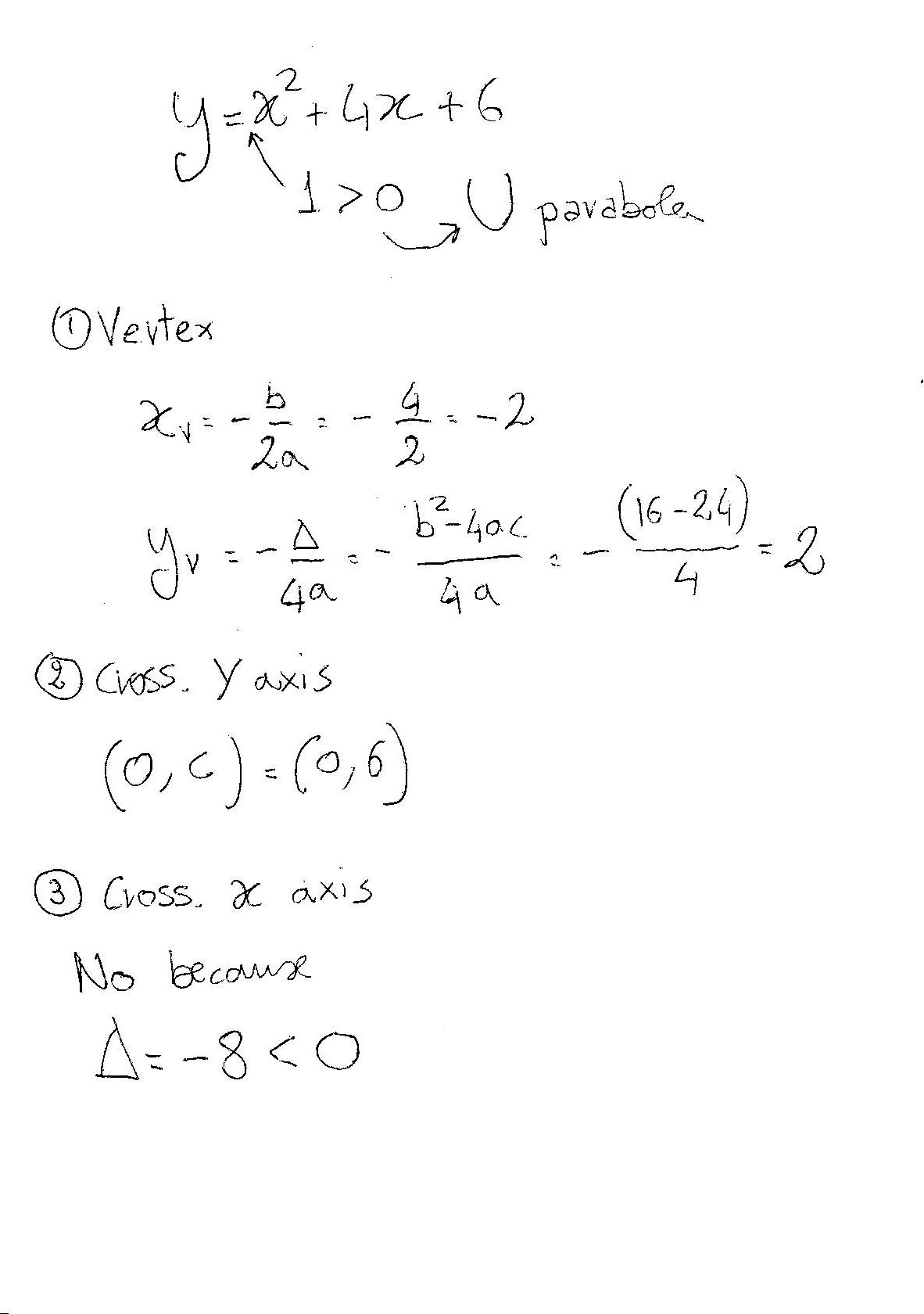



How Do I Graph The Quadratic Equation Y X 2 4x 6 Socratic
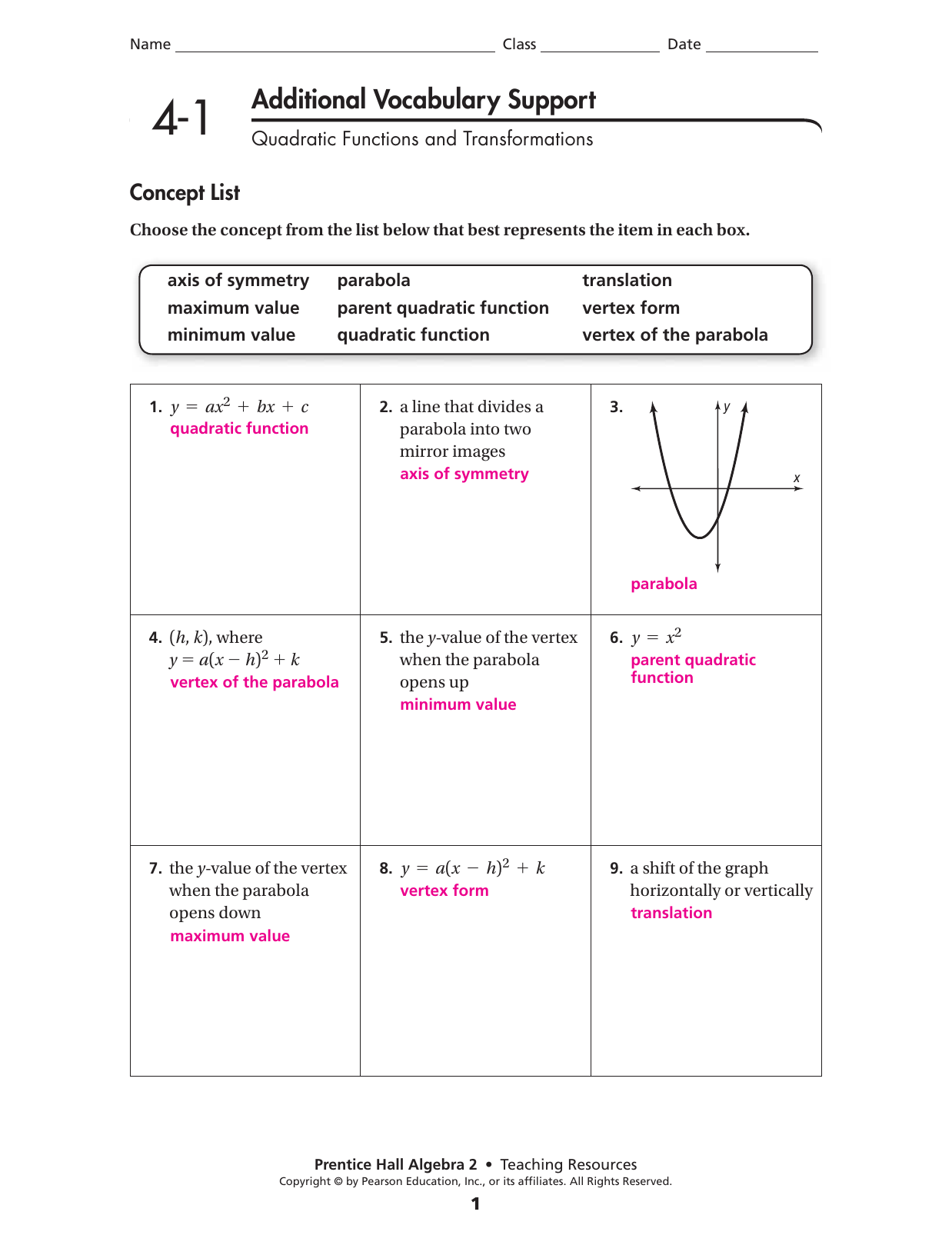



Additional Vocabulary Support Manualzz




Vertex Form Of Parabolas Flip Book Pages 1 4 Pubhtml5




Write The Quadratic Function In Standard Form F X Chegg Com
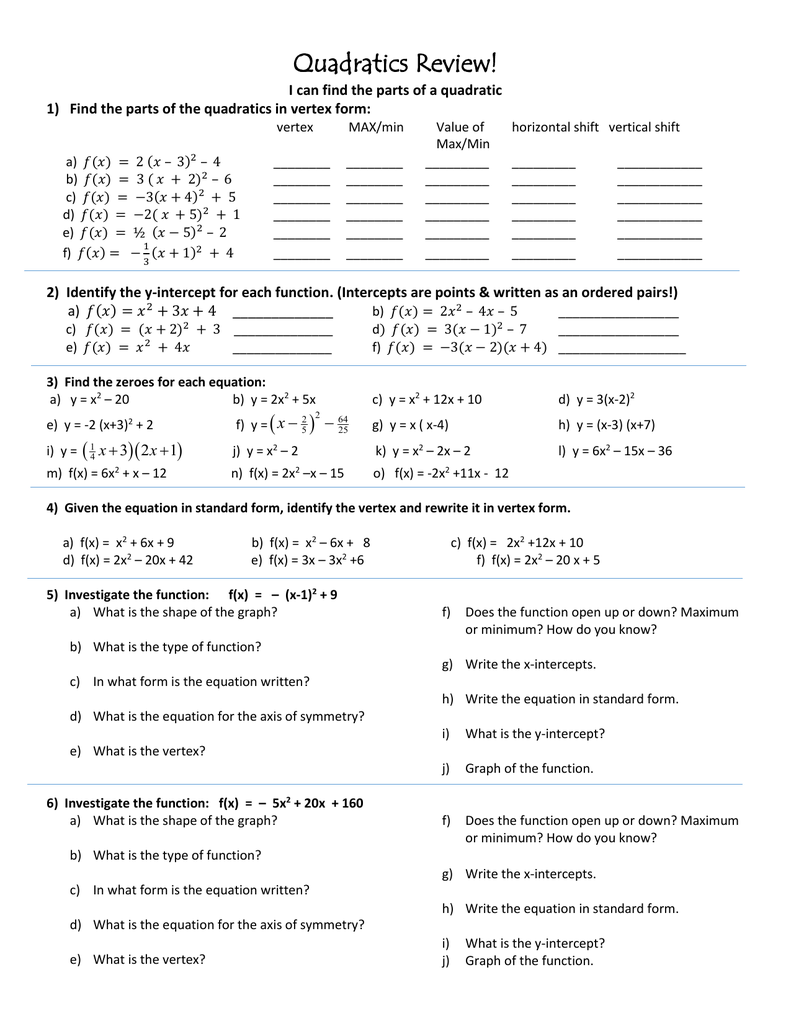



Quadratics Review 1 Quadrtics Test Review 1
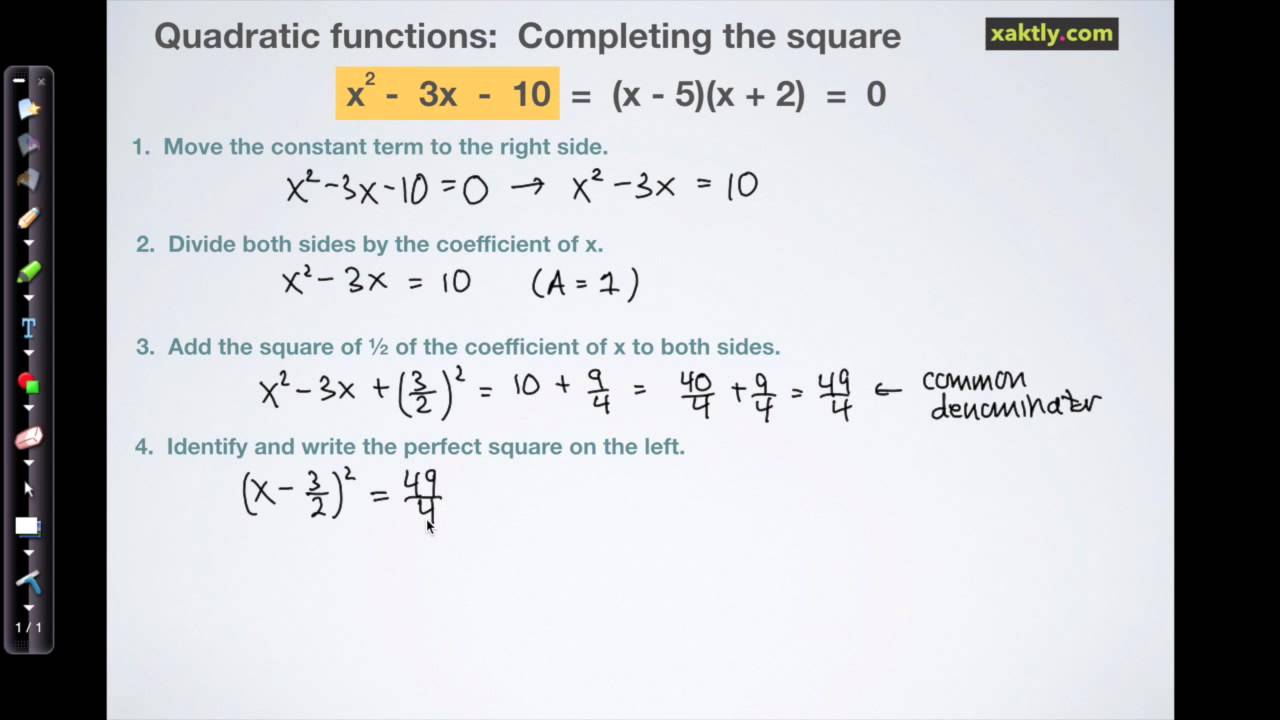



Quadratic Functions




Quadratic Functions Vertex Axis Of Symmetry Ppt Download




Find Factored Form And Vertex Of F X 2x 2 4x 6 Youtube




Ppt Completing Square Of A Quadratic Function Powerpoint Presentation Id
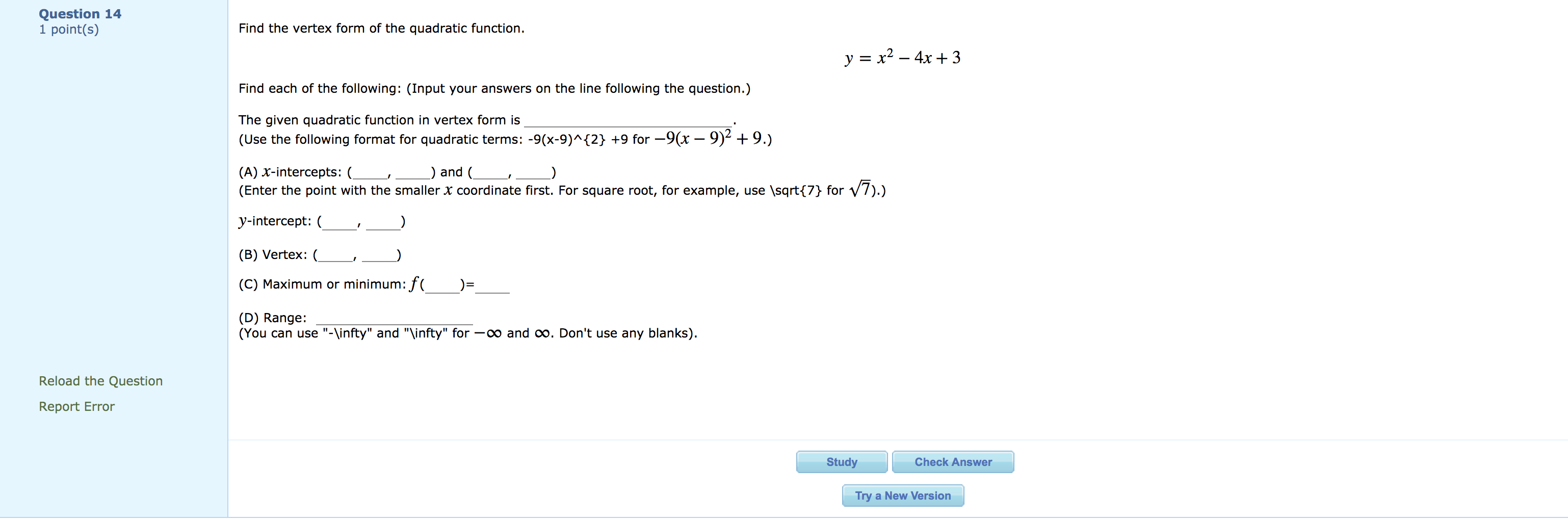



Solved Question 14 1 Point S Find The Vertex Form Of The Chegg Com




Converting Quadratics Vertex Form To Standard 13 Write An Equation For A Parabola With Vertex Pdf Document




Write Y 3x 2 24x 46 In Vertex Form Brainly Com




8 6 Practice Factoring To Solve Quadratic Equations Form G



Quadratic Functions



2 2 Characteristics Of Quadratic Functions Flip Ebook Pages 1 10 Anyflip Anyflip




7 1 Graphs Of Quadratic Functions In Vertex Form Quadratic Flip Ebook Pages 1 39 Anyflip Anyflip




How To Graph A Quadratic Equation 10 Steps With Pictures




Find The Axis Of Symmetry 1 And The Vertex 2 Of A Parabola Ppt Download




Step To Draw Y X 2 4x 1and Find Solution To Quadratic Equation Y X 2 5x 4 Youtube




Graph Quadratic Functions In Vertex Or Intercept Form Lesson Ppt Download



Connecting Everything Ppt Download




Finding Features Of Quadratic Functions Video Khan Academy




Vertex Form Of Parabolas Worksheet Kuta Software Llc Pages 1 4 Flip Pdf Download Fliphtml5



How To Write F X In Vertex Form




Quadratic Functions




Vertex Axis Of Symmetry Of A Parabola Video Khan Academy
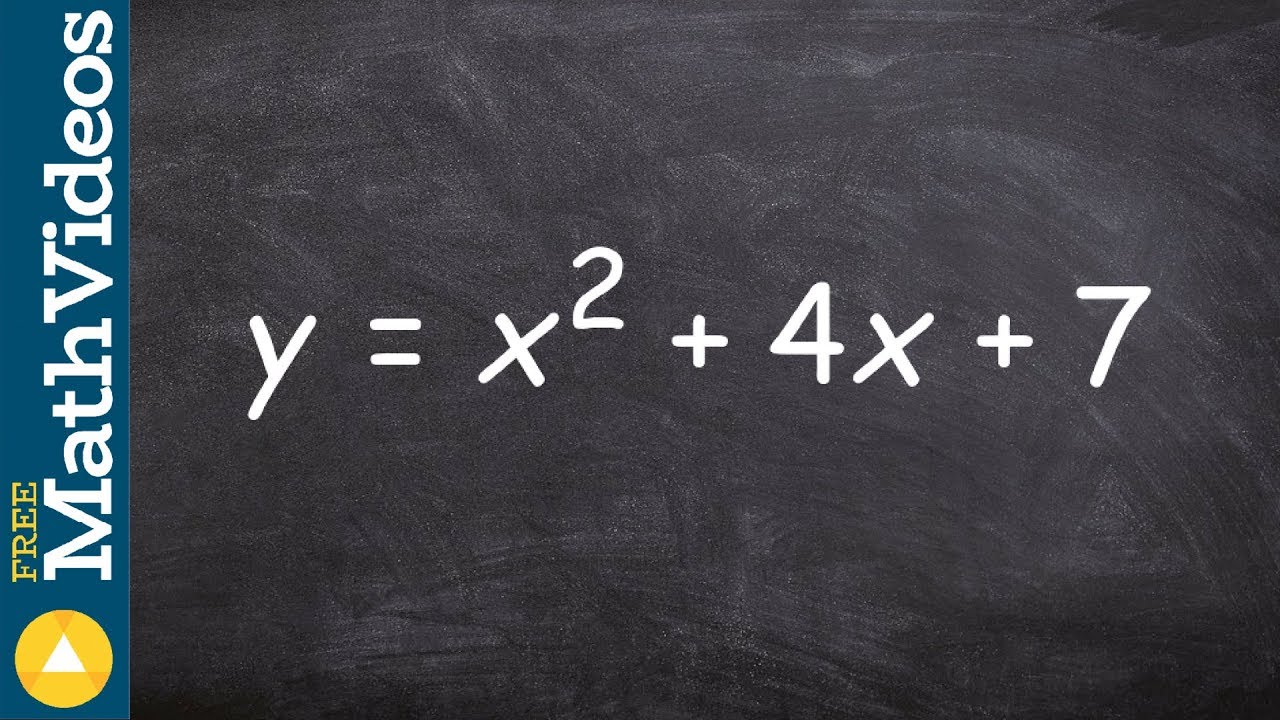



Learn How To Convert To Vertex Form By Completing The Square And Then Graph Y X 2 4x 7 Youtube



0 件のコメント:
コメントを投稿